1. 引言
近年来,带有高斯白噪声扰动的种群模型受到广泛关注,并取得了丰富的研究成果 [1] - [6]。而现实世界中,还存在着其它噪声,其中一些可能使种群系统存在状态随机切换,还有一些可能使种群数量在短时间内发生巨大变化,更合理的种群模型还应包括这些随机因素 [7] - [15]。特别地,在自然界中,种群之间的互惠关系是非常普遍的,许多学者对各种互惠种群模型进行了深入研究 [16] - [29]。
为探讨各种噪声对互惠种群模型的动力学行为的综合影响,受文献 [14] [15] [29] 的启发,本文考虑下列带Lévy跳的随机混杂互惠系统:
(1.1)
其中
表示第l个种群在时刻t的密度,
表示
的左极限;
是定义在带流概率空间
上的二维标准Brown运动,r是状态空间为S的连续时间Markov链;N是特征测度
在
的可测子集Y上满足
的Poisson计数测度,
是其补偿测度。对任意
,
,
为高斯白噪声的强度;函数
有界可测且
;
为正常数,相应的生物意义参见文献 [18]。
我们指出,与系统(1.1)对应的确定性自治模型最早由Graves等人 [18] 提出并研究,向等人 [19] 则考虑了相应的确定性非自治模型;仅包含高斯白噪声的情形首先由吕 [20] 研究,郭和丁 [21] 则讨论了其相应的非自治形式。据我们所知,关于系统(1.1)的研究还未见相关报道。本文旨在利用随机微分方程理论 [30] [31] [32],探讨系统(1.1)的正解的全局存在唯一性、随机持久性、灭绝性和平均意义下的持续性。
本文后续内容安排如下:第2节,给出一些准备工作;第3节,证明正解的全局存在唯一性;第4节,建立系统的随机持久性;第5节,讨论灭绝性和平均意义下的持续性;最后,数值模拟验证理论结果的合理性。
2. 准备工作
本节介绍一些定义、引理、假设和记号。
为方便讨论,给出以下记号:
1)
;
2)
,
,
;
3)
,
,
。
设
是带流完备概率空间,其中流
满足通常条件。Markov链
的状态空间
,其生成元
由
给出。其中,
,
是从i到j的转移速率,并且
。本文假定随机过程r,N和
是相互独立的,并且对任意的
,有
。因此,Q不可约,r是遍历的Markov链,Q存在唯一的不变分布
满足
及
,
,
。
考虑线性方程
(2.1)
其中
为列向量。
引理2.1. ( [30], p. 363) 下列断言成立:
1) 方程(2.1)有解的充要条件是
。
2) 若
和
是(2.1)的两个解,则存在
使得
,其中
为m个元素全为1的列向量。
3) 方程(2.1)的任意解可以表示成
,其中
是任意常数,
是方程(2.1)满足
的唯一解。
再考虑带有Lévy跳和Markov切换的随机微分方程:
(2.2)
其中
若对任意
,函数
关于t连续可微,关于x二次连续可微,则由Itô公式可知
其中
下面给出随机最终有界、随机持久、灭绝以及平均意义下持续的定义。
定义2.1. 若对任意
,存在正常数
,使得对初值
,系统(1.1)的解
满足
则称系统(1.1)的解是随机最终有上界的。
定义2.2. 若对任意的
,存在正常数
,使得对初值
,系统(1.1)的解
满足
则称系统(1.1)的解是随机最终有下界的。
定义2.3. 如果系统(1.1)的解既随机最终有上界又随机最终有下界,则称系统(1.1)是随机持久的。
定义 2.4. 设
是系统(1.1)的正解,
。
1) 若
a.s.,则称种群
是灭绝的;
2) 若
a.s.,则称种群
在平均意义下是非持续的;
3) 若
a.s.,则称种群
在平均意义下是强持续的。
下面是带有Lévy跳的指数鞅不等式。
引理 2.2. ( [31], p. 291) 设
为
-适应可料过程,若对任意
满足
则对任意
,有
3. 正解的全局存在性与唯一性
本节建立系统(1.1)的全局正解的存在唯一性,这是本文后续工作的基础。
定理3.1. 对任意初值
,系统(1.1)存在唯一的全局解
,并且该解以概率1停留在
中。
证明:易知系统(1.1)的系数满足局部Lipschitz条件,由随机微分方程解的存在唯一性定理可知,系统(1.1)在区间
上存在唯一的局部解
,其中
是爆破时刻。下面证明
是全局
的,即证明
几乎必然成立。取充分大的正整数
,使
和
。对任意
正整数
,定义停时:
对于空集
,规定
。易知
是一个单调递增序列。令
,则
。若证明
,则
。
下面用反证法证明
几乎必然成立。若该结论不成立,则存在常数
和
,使得
从而存在正整数
,对任意正整数
,有
(3.1)
定义Lyapunov函数:
显然,对任意
,
。由Itô公式可得
(3.2)
其中
(3.3)
记
则M为正常数。对(3.2)两边从0到
积分,然后取数学期望,再结合(3.3)可得
(3.4)
对
,记
。由
的定义可知,对每个
,
和
中至少有一个等于k或1/k。由(3.1)和(3.4)可知
其中
是
的示性函数。令
,可得
矛盾。所以
几乎必然成立。证毕。
4. 随机持久性
本节利用系统(1.1)的解的矩估计,证明其解的随机最终有界性,并进而得到系统的随机持久性。
引理4.1. 对任意
,存在正常数
,使得对任意初值
,系统(1.1)的解
满足
(4.1)
证明:由定理3.1知,对于任意初值
,系统(1.1)存在全局唯一解
,且以概率1停留在
中。定义Lyapunov函数
由Itô公式可得
(4.2)
其中
(4.3)
记
由Bernoulli不等式可知
从而
(4.4)
易知,
是与p有关的正常数。对(4.4)两边从0到t积分,然后取数学期望,再利用(4.2)和(4.3)可得
从而
令
可得
对
的情况,同理可证。证毕。
定理4.1. 系统(1.1)的解是随机最终有上界的。
证明:记
,对任意
,令
,由Chebyshev不等式可得
结合引理4.1中的(4.1)可得
从而
证毕。
下面证明系统(1.1)的解是随机最终有下界的。为此,令
(4.5)
由Itô公式可得
(4.6)
引理4.2. 若
,则对任意充分小的
,存在正常数L,使得对任意初值
,
,系统(1.1)的解
满足
(4.7)
证明:由(4.5),只需证明
注意到
由引理2.1可知方程
有解
。因此
取
,使得对每一
,有
定义Lyapunov函数
由(4.6)可得
(4.8)
根据生成元Q的性质可知
(4.9)
由Bernoulli不等式可知
 (4.10)
(4.10)
将(4.9)和(4.10)代入(4.8)可得
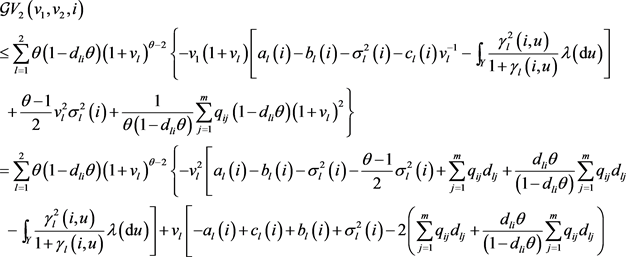
 (4.11)
(4.11)
取常数 ,使得对任意的
,使得对任意的 ,有
,有
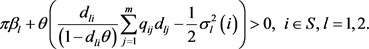
再取充分小常数 ,使得
,使得
 (4.12)
(4.12)
记
 (4.13)
(4.13)
由(4.12)易知,H是与 有关的正常数。由(4.11)和(4.13)可得
有关的正常数。由(4.11)和(4.13)可得
 (4.14)
(4.14)
由Itô公式,并结合(4.14)可知

从而
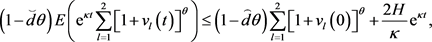
其中
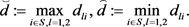
因此

令 可得
可得
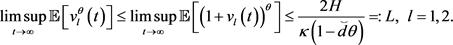
证毕。
定理4.2. 若 ,则系统(1.1)的解是随机最终有下界的。
,则系统(1.1)的解是随机最终有下界的。
证明:对任意 ,令
,令 ,由Chebyshev不等式可得
,由Chebyshev不等式可得
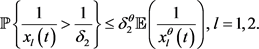
结合引理4.2中的(4.7)可得
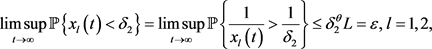
从而
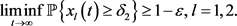
证毕。
联合定理4.1与定理4.2即得
定理4.3. 若 ,则系统(1.1)是随机持久的。
,则系统(1.1)是随机持久的。
5. 灭绝性与平均意义下的持续性
本节讨论系统(1.1)的灭绝性和平均意义下的持续性。为此,先利用引理2.2建立如下引理。
引理 5.1. 对任意初值 ,系统(1.1)的解
,系统(1.1)的解 满足
满足
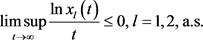
证明:对任意 ,由Itô公式可知
,由Itô公式可知
 (5.1)
(5.1)
注意到对任意 和
和 ,有
,有
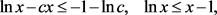
由(5.1)可知
 (5.2)
(5.2)
根据引理2.2,对任意 ,有
,有
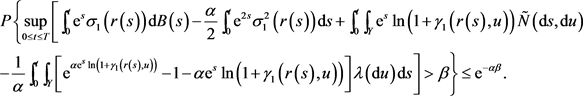
取 ,其中
,其中 。因为
。因为 ,根据Borel-Cantelli
,根据Borel-Cantelli
引理,存在 满足
满足 ,使得对任意
,使得对任意 ,存在正整数
,存在正整数 ,当
,当 时,成立
时,成立
 (5.3)
(5.3)
由Bernoulli不等式可知
 (5.4)
(5.4)
(5.2)式两边同除 ,再利用(5.3)和(5.4)可知,对任意
,再利用(5.3)和(5.4)可知,对任意 ,当
,当 ,
, 时,有
时,有
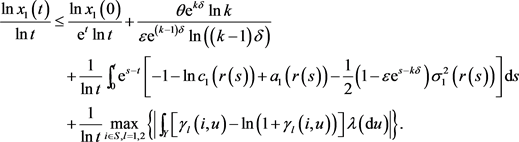
令 可得
可得
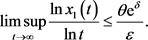
再令 ,可得
,可得
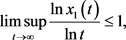
从而

对 的情况,同理可证。证毕。
的情况,同理可证。证毕。
下面依次给出灭绝性、平均意义下的非持续性和强持续性。
定理5.1. 对任意初值 ,系统(1.1)的解
,系统(1.1)的解 满足
满足
 (5.5)
(5.5)
特别的,如果 ,则种群
,则种群 趋于灭绝。
趋于灭绝。
证明:记
 。
。
由定理3.1知,对于任意初值 ,系统(1.1)存在全局唯一解
,系统(1.1)存在全局唯一解 ,且以概率1停留在
,且以概率1停留在 中。由Itô公式可得
中。由Itô公式可得
 (5.6)
(5.6)
其中
 和
和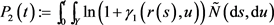
是局部平方可积鞅,并且
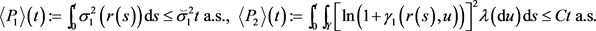
根据局部鞅的大数定律 [32] 可知
 (5.7)
(5.7)
(5.6)式两边同除t,然后取上极限,再利用(5.7)和Markov链 的遍历性可得
的遍历性可得
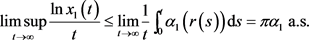
同理可知

特别的,若 ,则有
,则有
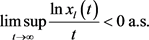
从而
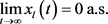
即种群 趋于灭绝。证毕
趋于灭绝。证毕
定理5.2. 若 ,则种群
,则种群 在平均意义下非持续。
在平均意义下非持续。
证明:若 ,由Markov链
,由Markov链 的遍历性可知
的遍历性可知
 (5.8)
(5.8)
任给 ,由(5.7)和(5.8)可知,存在正数T,对任意
,由(5.7)和(5.8)可知,存在正数T,对任意 ,有
,有
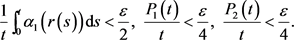
代入(5.6)可知,对任意 ,有
,有
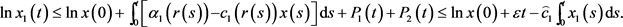
设 ,则对任意
,则对任意 ,有
,有
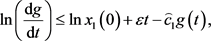
或者
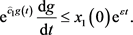
两边从T到t积分可得
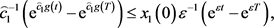
从而
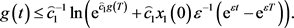
两边同除t,再取上极限可得
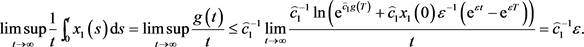
由 的任意性,可知
的任意性,可知
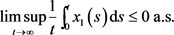
从而
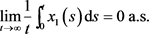
同理可证,若 ,则
,则
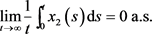
证毕。
定理5.3. 若 ,则种群
,则种群 在平均意义下强持续。
在平均意义下强持续。
证明:由Itô公式可知
 (5.9)
(5.9)
两边同除t,并移项可得
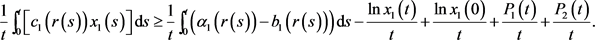
两边取下极限,并利用引理5.1和(5.7)式可得
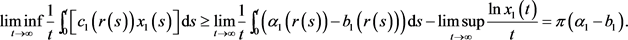
若 ,则
,则

对 的情况,同理可证。证毕。
的情况,同理可证。证毕。
6. 数值模拟
为验证理论分析结果,本节采用Milstein方法 [33] 对系统(1.1)进行数值模拟。
例6.1 在系统(1.1)中,设Markov链 的状态空间
的状态空间 ,生成元
,生成元
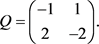
易知Q存在唯一不变分布
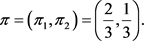
给定初值 ,且
,且 ,其它系数取值如下:
,其它系数取值如下:
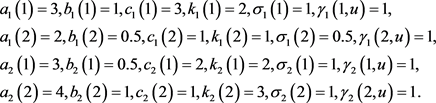
经简单计算可知
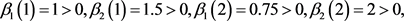
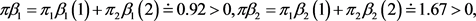
满足定理4.3的条件。从图1可知系统(1.1)是随机持久的。
例6.2 在系统(1.1)中,设Markov链 的状态空间
的状态空间 ,生成元
,生成元
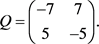
易知Q存在唯一不变分布
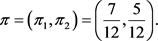
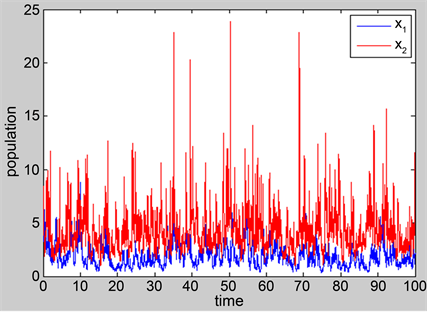
Figure 1. A solution of system (1.1) with 
图1. 当 时系统(1.1)的解
时系统(1.1)的解
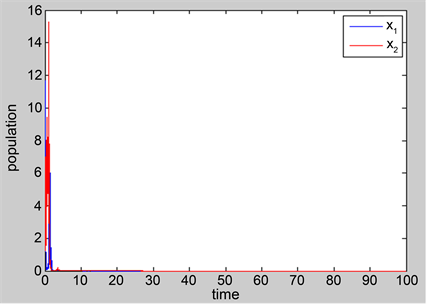
Figure 2. A solution of system (1.1) with 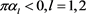
图2. 当 时系统(1.1)的解
时系统(1.1)的解
给定初值 ,且
,且 ,其它系数取值如下:
,其它系数取值如下:
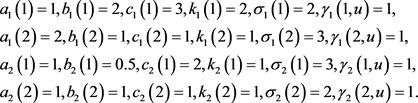
经简单计算可知
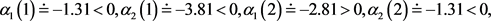
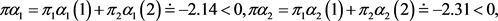
满足定理5.1的条件。从图2可知系统(1.1)是灭绝的。
基金项目
本文得到国家自然科学基金项目(11271110)和河南省教育厅科技攻关项目(15A120009)的支持。
NOTES
*通讯作者。