大约瑟夫逊结量子比特中拉比振荡的几何相位

Geometric Phase of Rabi Oscillations in a Large Josephson-Junction Qubit

摘要: 通过使用Lewis-Riesenfeld不变量理论，我们研究了大约瑟夫逊结量子比特中拉比振荡的几何相位。发现几何相位与偏置电流的直流和微波脉冲无关，也与微波的转换频率无关。

Abstract: By using the Lewis–Riesenfeld invariant theory, we have studied the geometric phase of Rabi oscillations in a large Josephson-junction qubit. The geometric phase has nothing to do with the dc and microwave pulses of bias current, and is independent of the transitions frequencies of microwaves.

1. 引言

1984年Berry研究发现，在绝热过程中量子力学波函数存在一个不可积的具有几何性质的相位因子，它不同于通常的动力学相位因子。这一发现在许多物理领域的几何相位因子已被广泛研究和证实，并逐渐通过相关实验证实。在最近20多年里，几何相位因子的研究已经成为量子力学中最重要的基本问题之一，其基本概念几乎渗透到物理学的各个领域 [1] - [31] 。本文将通过使用Lewis-Riesenfeld不变理论，研究大约瑟夫逊结量子位中拉比振荡的几何相位。

2. 物理模型

大约瑟夫逊结量子比特中拉比振荡的哈密顿算子可以写为 [32]

$\hat{H}={\hat{\sigma }}_x{I}_{\mu \omega c}\left(t\right)\sqrt{\hslash /2{\omega }_{10}C}/2+{\hat{\sigma }}_y{I}_{\mu \omega s}\left(t\right)\sqrt{\hslash /2{\omega }_{10}C}/2+{\hat{\sigma }}_z\frac{\delta I_{dc}\left(t\right)}{2}\frac{\partial E_{10}}{\partial I_{dc}},$ (1)

其中 ${\hat{\sigma }}_{x,y,z}$ 是泡利算符。用dc和微波脉冲的偏置电流可以完全操作量子位状态。是量子位状态之间的跃迁频率，C是结电容。此时

$I\left(t\right)=I_{dc}+\delta I_{dc}\left(t\right)+I_{\mu \omega c}\left(t\right)\cos {\omega }_{10}t+I_{\mu \omega s}\left(t\right)\sin {\omega }_{10}t,$ (2)

为方便期间，我们令

$A\left(t\right)=I_{\mu \omega c}\left(t\right)\sqrt{\hslash /2{\omega }_{10}C}/2,B(t)=I_{\mu \omega s}\left(t\right)\sqrt{\hslash /2{\omega }_{10}C}/2,C\left(t\right)=\frac{\delta I_{dc}\left(t\right)}{2}\frac{\partial E_{10}}{\partial I_{dc}}.$ (3)

方程(1)式变为

$\hat{H}=A\left(t\right){\hat{\sigma }}_x+B\left(t\right){\sigma }_y+C\left(t\right){\hat{\sigma }}_z.$ (4)

引入 ${\sigma }_{\pm }=\frac{1}{2}\left[{\sigma }_x\pm i{\sigma }_y\right]$ ，有

$\hat{H}=D\left(t\right){\hat{\sigma }}_{+}+D\left(t\right){\hat{\sigma }}_{-}+C\left(t\right){\hat{\sigma }}_z.$ (5)

其中 $D\left(t\right)=A\left(t\right)-iB\left(t\right)$ ，并且满足以下关系

$\left[{\hat{\sigma }}_{+},{\hat{\sigma }}_{-}\right]={\hat{\sigma }}_z,\left[{\hat{\sigma }}_z,{\hat{\sigma }}_{+}\right]=2{\hat{\sigma }}_{+},\left[{\hat{\sigma }}_z,{\hat{\sigma }}_{-}\right]=-2{\hat{\sigma }}_{-}.$ (6)

3. 动力学相位与几何相位

为了自洽，我们首先简要介绍Lewis-Riesenfeld(L-R)不变理 [27] 。对与时间有关的一维系统的哈密顿量 $\hat{H}\left(t\right)$ ，如果存在不变量运算符 $\hat{I}\left(t\right)$

$i\frac{\partial \hat{I}\left(t\right)}{\partial t}+\left[\hat{I}\left(t\right),\hat{H}\left(t\right)\right]=0.$ (7)

则可以给出与时间有关的不变量 $|{\lambda }_n,t\rangle$ 的特征值方程

$\hat{I}\left(t\right)|{\lambda }_n,t\rangle ={\lambda }_n|{\lambda }_n,t\rangle ,$ (8)

当 $\frac{\partial {\lambda }_n}{\partial t}=0$ ，此系统有时间有关的薛定谔方程是

$i\frac{\partial {|\psi \left(t\right)\rangle }_s}{\partial t}=\hat{H}\left(t\right){|\psi \left(t\right)\rangle }_s.$ (9)

根据L-R不变理论，方程(9)的特定解 ${|{\lambda }_n,t\rangle }_s$ 只有相位因子 $\exp \left[i{\delta }_n\left(t\right)\right]$ 与 $\hat{I}\left(t\right)$ 的本征函数 $|{\lambda }_n,t\rangle$ 不同，即

${|{\lambda }_n,t\rangle }_s=\exp \left[i{\delta }_n\left(t\right)\right]|{\lambda }_n,t\rangle ,$ (10)

这表明 ${|{\lambda }_n,t\rangle }_s\left(n=1,2,\cdots \right)$ 构成方程(9)解的完整集合。那么薛定谔方程(9)的一般解可以写成

${|\psi \left(t\right)\rangle }_s={\displaystyle \underset{n}{\sum }{C}_n}\exp \left[i{\delta }_n\left(t\right)\right]|{\lambda }_n,t\rangle ,$ (11)

其中

${\delta }_n\left(t\right)={\displaystyle {\int }_0^t\text{d}{t}^{\prime }}\langle {\lambda }_n,t^{\prime }|i\frac{\partial }{\partial t^{\prime }}-\hat{H}\left(t^{\prime }\right)|{\lambda }_n,t\rangle ,$ (12)

且 $C_n={\langle {\lambda }_n,0|\psi \left(0\right)\rangle }_s$ 。

对于由方程(5)哈密顿量描述的系统，我们可以定义以下不变量

$\hat{I}\left(t\right)=\alpha \left(t\right){\hat{\sigma }}_{+}+{\hat{\alpha }}^{*}\left(t\right){\hat{\sigma }}_{-}+\beta \left(t\right){\hat{\sigma }}_z.$ (13)

将方程(5)和(13)代入到方程(7)，可以得到一个辅助方程

$i\stackrel{\dot{}}{\alpha }\left(t\right)+2\left(D\beta -\alpha \beta \right)=0,i\stackrel{\dot{}}{\beta }+D\left(\alpha -{\alpha }^{*}\right)=0.$ (14)

为了获得一个与时间无关的不变量，我们可以引入么正变换算子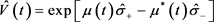，当满足以下关系式时

$\beta \cos \left(2\left|\mu \right|\right)+\alpha \mu \sqrt{2\left|\mu \right|}\left[\sin \sqrt{2\left|\mu \right|}+\frac{1}{\sqrt{2\left|\mu \right|}}-1\right]=1,$ (15)

$\alpha +\frac{\mu \left|\beta \right|}{\left|\mu \right|}\left[\sin \left(2\left|\mu \right|\right)+2\left|\mu \right|-1\right]=1,$ (16)

有

${\hat{I}}_V\equiv {\hat{V}}^{†}\left(t\right)\hat{I}\left(t\right)\hat{V}\left(t\right)={\hat{\sigma }}_z.$ (17)

通过使用Baker-Campbell-Hausdoff公式 [33]

${\hat{V}}^{†}\left(t\right)\frac{\partial \hat{V}\left(t\right)}{\partial t}=\frac{\partial \hat{L}}{\partial t}+\frac{1}{2!}\left[\frac{\partial \hat{L}}{\partial t},\hat{L}\right]+\frac{1}{3!}\left[\left[\frac{\partial \hat{L}}{\partial t},\hat{L}\right],\hat{L}\right]+\frac{1}{4}\left[\left[\left[\frac{\partial \hat{L}}{\partial t},\hat{L}\right],\hat{L}\right],\hat{L}\right]+\cdots ,$ (18)

且 $\hat{V}\left(t\right)=\exp \left[\hat{L}\left(t\right)\right]$ 。满足下面方程

$D+C+\frac{\beta \mu }{\left|\mu \right|}\left[\sin 2\left|\mu \right|+2\left|\mu \right|-1\right]+\frac{2\mu \left(\mu {\stackrel{\dot{}}{\mu }}^{*}-\stackrel{\dot{}}{\mu }{\mu }^{*}\right)}{{\left(2\left|\mu \right|\right)}^3}\left[\sin \left(2\left|\mu \right|-2{\left|\mu \right|}^3\right)\right]=0,$ (19)

有

${\hat{H}}_V\left(t\right)={\hat{V}}^{†}\left(t\right)\hat{H}\left(t\right)\hat{V}\left(t\right)-i{\hat{V}}^{†}\left(t\right)\frac{\partial \hat{V}\left(t\right)}{\partial t}=\lambda {\hat{\sigma }}_z,$ (20)

其中：

$\lambda =i\left[1-\cos \left(2\left|\mu \right|\right)\right]\left(\mu {\stackrel{\dot{}}{\mu }}^{*}-\stackrel{\dot{}}{\mu }{\mu }^{*}\right)+D\left[\sin \sqrt{2\left|\mu \right|}+\frac{1}{2\sqrt{2\left|\mu \right|}}-1\right]\sqrt{2\left|\mu \right|}\left[\alpha \mu +{\alpha }^{*}{\mu }^{*}\right],$ (21)

该量子系统具有的几何相位为：

${\stackrel{\dot{}}{\delta }}^g\left(t\right)=i\left[1-\cos \left(2\left|\mu \right|\right)\right]\left(\mu {\stackrel{\dot{}}{\mu }}^{*}-\stackrel{\dot{}}{\mu }{\mu }^{*}\right).$ (22)

4. 结论

在本文中，我们研究了大约瑟夫逊结量子比特中拉比振荡的几何相位。发现几何相位与偏置电流的直流和微波脉冲无关，也与微波的转换频率无关。很明显，几何相具有纯粹的几何和拓扑特征。这种几何因子是一种新的量子效应，且体现在介观电路中。我们期待该结论在将来的实验中得以证实。

基金项目

本文得到北京信息科技大学研究生院基金支持。