1. 引言
目前为止,研究人员们提出并发展了很多求解偏微分方程的方法,如Lie对称 [1] [2] 、Adomian分解法 [3] 、同伦摄动法 [4] [5] 、同伦分析法 [6] 、精确解方法 [7] [8] 等等。这些方法中,Adomian分解法(Adomian Decomposition Method-ADM)是由美国数学物理学家Georgie Adomian [3] 教授提出并发展起来的一种方法。该方法中假定方程的因变量和非线性项都能分解为级数。非线性项的分解式被称为Adomian多项式,段 [9] [10] 研究了Adomian多项式的计算方法的优化。Cherruault [11] 等人研究了Adomian分解法的收敛性问题。Wazwaz [12] 基于Adomian分解法考虑了奇异初值问题;D. Lesnic [13] 基于Adomian分解法考虑了广义Boussinesq问题;朱永贵 [14] 给出了Adomian逆算符方法,通过实现该算法的算例,验证了Adomian分解法的有效性。
Shidfar [15] 和Patel [16] 等人基于Adomian分解法针对矩形区域对应的偏微分方程的(初)边值问题进行研究,并提出了不同的算法。我们 [17] 对矩形区域对应的偏微分方程的(初)边值问题提出了分段带权Adomian分解法。
本文中基于Adomian分解法考虑三角形区域对应的偏微分方程的边值问题,即三角形地下水流区域上的异质含水层模型。地下水流区域上的异质含水层模型控制微分方程为:
 (1)
(1)
其中 是水头函数
是水头函数 ;
; 表示月平均降雨补给
表示月平均降雨补给 ;
; 表示含水层渗透系数
表示含水层渗透系数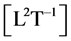 。设附加边界条件为
。设附加边界条件为
 (2)
(2)
斜边 上:
上:
 (3)
(3)
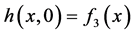 (4)
(4)
其中 ,
, ,
, 。
。
2. 地下水流区域上的异质含水层模型的分段Adomian近似解
将方程(1)改写成
 (5)
(5)
其中, 。
。
步骤1:利用直线 与
与 先将区域
先将区域 划分成如下三块,如图1所示
划分成如下三块,如图1所示
 (6)
(6)
步骤2:在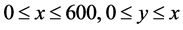 上,在方程(5)两侧作用于
上,在方程(5)两侧作用于

考虑边界条件 ,并令
,并令 后得到
后得到
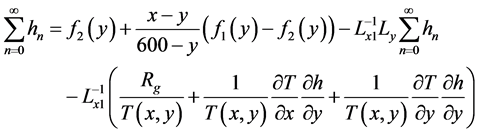 (7)
(7)
将函数 在原点Taylor展开,并记为:
在原点Taylor展开,并记为: 其中
其中
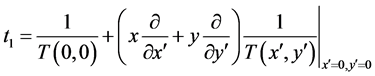

那么,有
 (8)
(8)
 (9)
(9)
由此,根据(7)-(9)构造循环公式,如下:
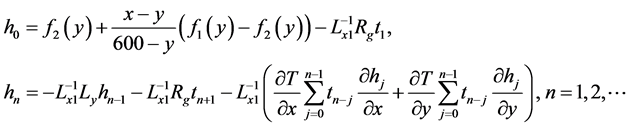
从这个循环公式得到
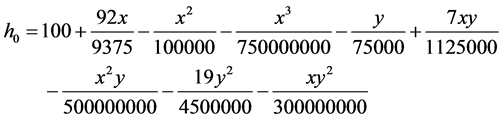
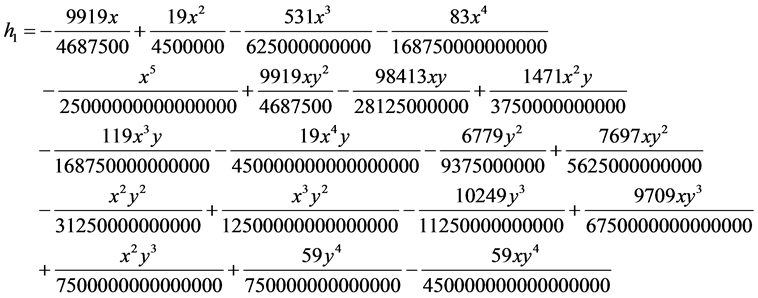
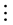
从而得到方程(5)与 的
的 项Adomian近似解
项Adomian近似解
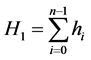
步骤3:在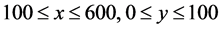 上,在方程(5)两边作用于算子
上,在方程(5)两边作用于算子
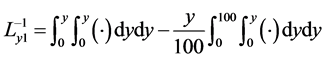
同时考虑边界条件 ,
, ,并令
,并令 后得到
后得到
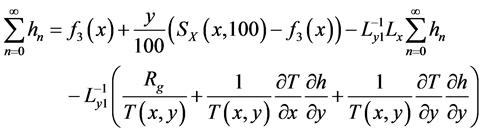
根据(8)-(9),构造循环公式
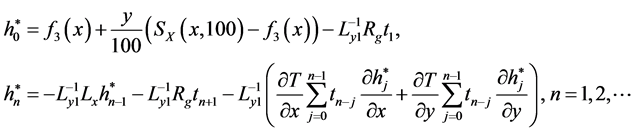
从这个循环公式得到(5)与 的
的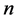 项Adomian近似解
项Adomian近似解
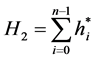
其中 。
。
步4:在方程(5)两侧作用于 ,同时考虑
,同时考虑
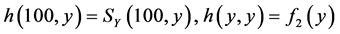
与公式(8)-(9),并令 后获得
后获得

从这个循环公式得到(5)与 的
的 项Adomian近似解
项Adomian近似解
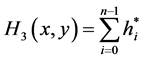
其中 。
。
最后归纳以上部分解得到整个区域上的分段近似解,如图2所示,
3. 结果分析
分段近似解 除了边界线
除了边界线 和
和 上的
上的 两小段边界以外,
两小段边界以外, 精确满足其余的所有的边界条件。为了表征
精确满足其余的所有的边界条件。为了表征 的近似程度,令边界误差为
的近似程度,令边界误差为
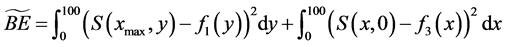
运用余函数来表示近似解在区域内任意一点处的误差
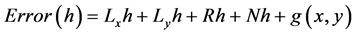
利用 表征近似解的方程误差,其中
表征近似解的方程误差,其中 是
是 范数。本文中得到的三项分段近似解
范数。本文中得到的三项分段近似解 的边界误差和方程误差分别为
的边界误差和方程误差分别为 ,
, 。
。
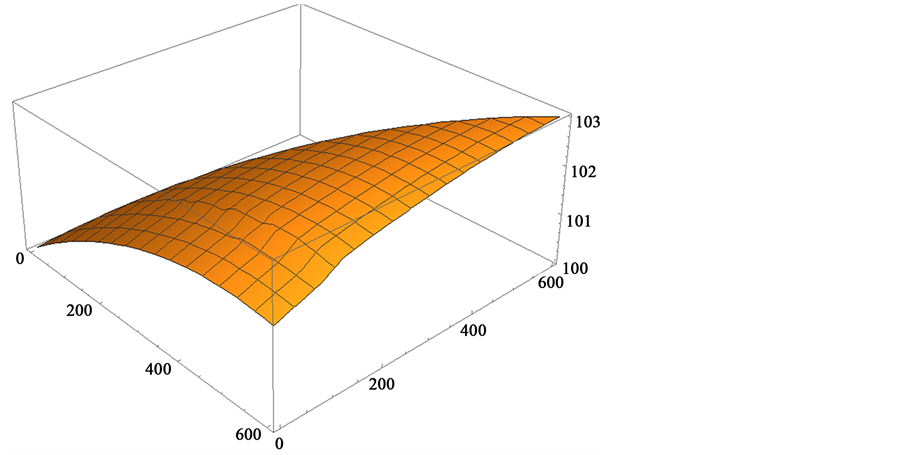
Figure 2. Segmented three-terms approximate solution 
图2. 分段三项近似解
基金项目
内蒙古自治区面向基金项目(2016MS0109),内蒙古自治区高等学校科研研究项目(NJZY094),内蒙古工业大学重点项目(ZD201515)。
NOTES
*通讯作者。